

 Vol. 39 (Number 46) Year 2018. Page 12
Vol. 39 (Number 46) Year 2018. Page 12
Sergey Viktorovich SHCHERBATYKH 1; Kseniya Gennadyevna LYKOVA 2
Received: 30/05/2018 • Approved: 14/07/2018
ABSTRACT: The article shows that implementation of the provisions of the continuity and incessancy principles together with integrated application of information and remote technologies, as well as innovative means of assessment and evaluation allow one to identify positive dynamism in measuring the levels of the stochastic competence formedness in high school students. High performance can be achieved by means of a proper combination of these teaching and assessment means and methods, identification of underlying intrasubject and interdisciplinary genetic affinity of a subject, selection of a set of real-world problems that would allow the competence to manifest itself in full |
RESUMEN: El artículo muestra que la implementación de las disposiciones de los principios de continuidad e incesancia, junto con la aplicación integrada de información y tecnologías remotas, así como los medios innovadores de evaluación, permiten identificar un dinamismo positivo en la medición de los niveles de competencia estocástica en niveles altos de estudiantes de escuela. Se puede lograr un alto rendimiento por medio de una combinación adecuada de estos medios y métodos de enseñanza y evaluación, la identificación de intrasujetos subyacentes y la afinidad genética interdisciplinaria de un sujeto y la selección de un conjunto de problemas del mundo real que permitirían que la competencia se manifieste en sí misma de manera plana. Palabras clave: competencia estocástica, continuidad, incesancia, preparación para la instrucción, tecnologías de la información. |
In modern information society, it is not only knowledge that is valued as never before, but also the ability to apply, update and improve it, that is, to form competences in school students. This is a key element not only for each person’s creative self-fulfillment, but also for a global development of society. According to requirements imposed by state educational standards and social mandate, as well as personal expectations of school students in the education system, there has been a shift in paradigms expected to form competences in students. In this respect, it is growing more urgent to search and develop a methodical concept that could meet the requirements and create favorable conditions for development of the intellectual potential in students.
The purpose of the study: development and substantiation of a stochastic competence formation concept based on a principle of continuity as the major factor in effective learning process organization.
The issue of developing the most effective and successful teaching methods that would guarantee a high personal performance of school students has remained relevant for education throughout the entire period of its formation. Reforms in the modern educational system have led to identification of such a property as flexibility, that is, the ability to quickly adapt to changing demands of the social mandate and each stage of education against the previous one. The main component that makes it possible to implement the flexibility of education subsystems and interaction of their elements is the continuity principle.
The whole variety of society requirements for future graduates determines in their school days the developing ability to possess, receive and apply information, to analyze and critically assess situations. Therefore, special attention should be paid to solid stochastic knowledge formation in general educational development of a student’s personality, which plays an important role in processing and perception of information about social, political, economic or other spheres of life (Shor, 1977: 21).
Modern education is entrusted with the task to develop such personal qualities in high school students that would prove useful to society in their socially significant activity, facilitate appreciation of the present-day worldview and methods of its research (Byvsheva, 2012: 28). It follows therefrom that in the context of school mathematical education it is important to form a stochastic competence as one of the components of productive student educational activity.
The continuity principle in formulating a methodological concept of the stochastic competence development in students of the 10th and 11th grades of general education schools will serve as a crucial component in increasing the overall effectiveness of the educational process, implementation of continuity, “incrementality” of the educational process, and quality training of future graduates at a higher level (Asmolov, 2016: 20). The evidence thereof affirms that this principle implementation possibility is becoming reasonably required.
The problem of stochastic competence formation in secondary school students was considered in a paper of I.V. Kitaeva (2017). However, this question statement research based on the principles of continuity and incessancy has not been studied before, and the comprehensiveness of integrating conventional and information methods as well as teaching and assessment means guarantees high performance.
An analytical review of literature on the continuity problem in education has shown that certain time periods are characterized by the fact that this issue has been either not discussed at all, or touched upon indirectly as a result of studying certain methodological principles of education and the modern education system development.
Since late 1917, following the nationalization of all types of educational institutions when schools became generally accessible and compulsory, special attention has been paid to continuity of all the education stages as a key factor that provides equal educational opportunities. One of the first references to the term “succession” can be found in the work of M.M. Pistrak “Pedagogy” (1934) as a general pedagogical principle of the education system that determines the “unity” of school characterized by the continuity of all its stages, that is, continuity is a link for school students to proceed from primary to secondary school, and from secondary to higher education. A number of scholars hold to a similar opinion that continuity is a general pedagogical principle that determines coherence and interrelationship, integrity and unity of the learning process at all stages of education (Godnik, 1990: 75).
Other researchers, such as N.N. Oleinik (1974), D. Sh. Sitdikova (1985), T.P. Payson (2007: 158), R.M. Zainichev (2015) and others tend to define continuity as a general pedagogical regularity expressed in successive relation “from one academic year to another” qualitatively increasing the educational process efficiency in terms of its quality (Smantzer, 2013: 63).
Such educators as A.A. Kyveryalg (1986), V.N. Revtovich (1987) understand continuity as a methodological principle of learning process at different stages of education as a universal basis for advance and development of student knowledge (Kyveryalg and Mikhailov, 1986: 74).
Such didacticians of the 1950s as P.N. Gruzdev (1940), I.T. Ogorodnikov and P.N. Shimbirev (1950) and others do not use the concept of “continuity” at all when considering didactic principle; however, when specifying certain issues, they imply constituent elements of continuity: systematicity, consistency, and perspectivity. Thus, P.N. Gruzdev’s works formulate systematicity and consistency as a synonym for continuity: acquiring knowledge, expertise and skills involves a certain sequence of the studied material accumulation that would rely on a previous sequence, while the previous one would be expanded and strengthened by the subsequent one, thereby ensuring the learning process integrity (Gruzdev, 1940: 281).
At the present stage of the education system development, the concept of continuity is being expanded and supplemented, becoming more “flexible” and adaptive:
• continuity implements a unified pedagogical tendency that contributes to a systematic, gradual and consistent implementation of the student personality learning and development process in ever-changing learning environment as a regular phenomenon of the educational process;
• a process of interaction, whereby new experience accounting for continuous development of the education system is formed based on past experience (Arkhipova, 2009),
• a process and a result of manifesting systematicity and continuity of academic programs acquisition, of the functioning system of learner’s continuous personal education (Oreshkina, 2003: 194);
• a process of progressive individual development at each stage of continuous education, depending on the genetic affinity of the stages of education and student development (Prosvirkin, 2010: 43).
Revealing the relevance of the issue under study, it should be noted that so far, there have been theoretical discrepancies in the approaches to solving the problem of continuity in education. It consists in an ambiguous approach to explaining continuity either as a general pedagogical regularity or as a general pedagogical or methodological principle. The latter in turn allows one to provide insight into “underlying” theoretical aspects of the intrinsic property of continuity in the modern education system, to identify a system of measures that would determine the purposefulness and systematic nature of genetic affinity in the educational process. As a result, the lack of a unifying pedagogical vision that would ensure functioning and realization of continuity in mathematical education is subject to further research and refinement.
The highest premium in the formation of stochastics as a science is set on such scholars as A.N. Kolmogorov (1938), A.Ya. Khinchin (1938), V.Ya. Bunyakovsky (1846), Ye.Ye. Slutsky (1960) and many others. Despite the discontinuity of its development, it is today when it is on the highest rise pursuant to its expansion and application in many sciences and technologies. Studying elements of the probability theory, combinatorial analysis and mathematical statistics in the secondary school course in mathematics makes it possible to reveal the universal character of stochastic methods used in widely different areas, to determine potential scope for their application in future professional activities (Concept of the development of mathematical education in the Russian Federation, 2013).
One of the strategic ideas of the modern education system is to create an environment that would meet the requirements of society, the government and the labor market to provide quality education as a result of designing new institutional mechanisms for regulating the education sector that would update the genetic affinity in teaching and improve the structure, content and practical focus of academic programs.
The most important factor in the learning process efficiency that allows for a smooth transition from one stage of education to another is properly formed expertise and skills of student information activity, the ability to apply knowledge in solving real-world problems, that is, to form competences. The framework for information provision for high school students in the school course in mathematics is a focus on the effective use of information resources, the latest technical means and software products of information technologies, various means of remote technologies that create optimal conditions for individual development and improvement of students for the period of their school career (Bunck et al., 2017: 293).
An important role in the research was played by a search for methodological problems of forecasting mathematical education in general and through the example of stochastics, a selection of the most optimal teaching and assessment methods and means for its successful implementation. The factor analysis the authors carried out has allowed them to explore the existing educational experience and draw theoretical inferences therefrom. Curricula and academic documentation of general education schools in Lipetsk Region (Russia), educational standards, thesis works on the subjects under consideration have been studied, and an online learning course on stochastics has been introduced into the educational process. The main findings and conclusions were obtained through the use of experimental and practical methods, such as questionnaire diagnostic technique (questionnaires, test administration, interviews), analysis of student activities in the 10th and 11th grades, observation and generalization of the experience. The experimental work was undertaken on the premises of general schools in Yelets, Lipetsk Region. The sample consisted of 10th and 11th grade students majoring in physics and mathematics as well as in humanities and social studies, whose training was conducted in accordance with the integrated approach provisions of teaching and assessment methods and means using the means of remote learning along with the conventional ones. In the control group, the learning process was standard. Quantitative analysis of the results obtained was carried out using statistical methods.
According to the concept of modernization, there is a shift in educational paradigms of the mathematical education system in school. As a result, special attention is not paid to primary objective results (fundamental knowledge of students), but to meta-subject results that are in charge of certain experience formation in high school students: learning and cognitive activity self-organization skills, the ability to make independent choices, to draw accurate conclusions and inferences, and to find their own well-reasoned position. Therefore, one of the priority directions in the math culture development within the educational process is the stochastic competence formation in future general education school leavers, whose success factors will be the principles of continuity and incessancy. Stochastic competence is the readiness and ability of students to apply the acquired stochastic knowledge, expertise and skills in solving real-world problems, including non-standard ones, both in mathematics and other disciplines that involve the skills of evaluation, selection, and processing of information. Possessing the stochastic competence will help students in further education in a higher educational institution and subsequent employment.
A methodical concept containing a theoretical system of natural science and technical knowledge aimed at identifying genetic affinity and learning continuity throughout the entire learning process is capable of developing the stochastic competence in school students.
It is possible to determine the level of the stochastic competence formedness in students by creating certain pedagogical situations that would allow for its fullest application. This evidence causes the issue of designing an environment that would allow the competence to exercise in the educational process.
A solution to the problem of designing an environment for continuous and successive development of high school students in general education schools is the developed concept of the stochastic competence measurement. It is based on the idea that modernization of the stochastic education system involves, among other things, a change in the evaluation of student progress in accordance with requirements imposed by the social mandate and the state, as well as personal expectations of high school students.
The statement about a necessity to use a set of interrelated assessment methods and means that gives an idea of the competence principles already inculcated in middle school used to determine a competence is a fundamental premise. Academic performance assessment makes it possible to establish the level of competence formedness developing due to the continuity of educational programs. The implementation of this provision is stipulated by a coordinated system of requirements of national educational standards, competency-oriented requirements for the quality of training of future graduates included in the set of conventional assessment methods and means used, as well as innovative indicators that do not reflect only a knowledge component but also a motivational activity component.
Quantitative assessment of development levels, identification of personal traits and qualities of students transforming in the process of education is an important value of pedagogical measurements.
The concept of continuity in measuring and evaluating the stochastic competence of school leavers contains the following provisions:
• the competency of a high school student is an indicator of the quality of all their educational performance results;
• the competence of graduates is revealed through a combined reproduction of previously reversed competences that meet the declared requirements imposed by the state, the social mandate, and the expectations of a student themselves;
• objective evaluation of competences in high school students is achieved through comprehensive monitoring of the main constituent elements both by conventional methods and means and by modern innovative ones. One can determine the level of competence formedness with the help of a cumulative performance recording system and a criteria-level approach to interpreting the overall school student performance;
• the content of mathematical competence is determined by the formedness level of the stochastic competence, whose foundations were laid in middle school and have developed driven by the principles of continuity and incessancy;
• the following are referred to the assessment and evaluation activities: preparation of assessment and measuring materials and definition of diagnosable indicators.
The factors accounting for implementation of the concept ideas are knowledge, expertise and skills of students at a previous stage of the educational process and their active conscious activity in the educational process both during class time and non-school hours through the use of information or remote learning technologies.
The following approaches are methodological and theoretical bases for the stochastic competence development:
• institutional approach is characterized by all structures and levels of education functionality and their coherence with other social institutions (family, science, etc.), thereby, providing mutually supportive relationship with each other;
• system approach: continuity is a succession and consistency of the content of education, methods and organizational forms of education, that is, reliance on the previous stage of education and its orientation to the subsequent one; at the same time, interrelationship between the activities of educational institutions;
• procedural approach: continuity is the process of continuous interaction based on past experience and the formation of a new one that modifies the education system and is in charge of personal development and education of a learner.
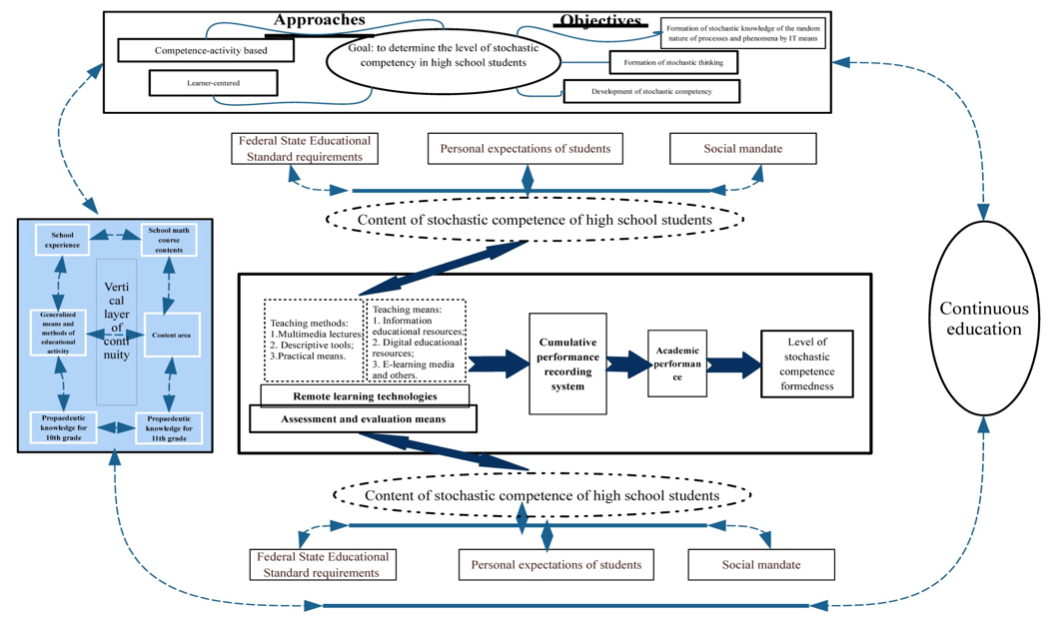
Based on this concept, a theoretical model for measuring the levels of the stochastic competence formedness in students of 10th and 11th grades of general education schools has been designed based on the principles of continuity and incessancy in teaching mathematics and establishing quality performance indicators (Figure 1).
A theoretical model is understood as a dynamically developing system, with vectored learning activity organized and regulated in the information space within its framework. This model is aimed at studying the obtained resulting data of a research object. When designing the model, one of the objectives was a comprehensive approach including external control (the Unified State Exam results) and internal control, that is, application of conventional assessment methods and means, as well as innovative indicators. The assessment activity results are the basis of a cumulative performance recoding system; they facilitate performance indicators calculation, the generalization of which brings one to determining the level of stochastic competence formedness as the final stage of successful curriculum completion.
The major purpose of control is to assess the quality of mathematical competence in future graduates.
Problems to be solved include:
• correlation of the educational performance of school leavers and requirements imposed by the state and the social mandate, as well as personal expectations of students;
• measuring the competence of school students and determining the level of its formedness at the final stage of instruction with subsequently ascertained qualification of graduates in terms of their professional becoming and self-identification.
The basic principles that determine the model functionality:
• the principles of incessancy and continuity, that is, consistency of all the educational process stages;
• the principle of integration, that is, a holistic view of a high school student competence as a result of its constituent modifications;
• the principle of comprehensiveness and complexity, that is, the competence evaluation from different perspectives, adjustment of its individual components and indicators;
• the principle of individualization - the organization of a learner-oriented educational process;
• the principle of fundamentality and applied orientation, that is, not only the presence of distinctly formed theoretical and practical knowledge in students, but also of interconnection of the two.
Figure 1
Model for measuring the stochastic competences in 10th and 11th grade students
• the principle of flexibility, that is, a rapid response to changes in a course contents, prompt adaptation of the course to changing requirements of educational paradigms (that is, effective educational structure transformation taking into account the application of a variety of options: multileveledness, multifunctionality, multistaging) (Kharisova, 2017: 58).
The model consists of the following interrelated and interdependent blocks: methodological, substantive, procedural and performance assessment.
The methodological block deals with identification of learning objectives, whereby students should develop a pre-planned stochastic educational background.
The substantive block determines basic elements of the stochastic competence content that meet the requirements of national educational standards and the social mandate. An invariant and a variable part have been distinguished within a competence structure, including the motivational, knowledge and activity components.
The procedural block exerts targeted pedagogical influence on stimulation of educational activities on the stochastic competence formation in high school students. It is based on different scientifically grounded teaching methods and means, remote learning technologies, control means that create favorable environment for the use of knowledge, skills and expertise of a cumulative system for recording control results that allow students to demonstrate their competence.
The performance assessment block deals with analyzing the learning efficiency, determines the level of the stochastic competence formedness with the help of criteria-level approach to evaluation of the received, predicted and pre-planned results of student activity (Volk et al., 2017: 17).
Within the scope of this theoretical model, a methodology has been developed for assessing the level of the stochastic competence formedness in high school students of general education schools based on a comprehensive approach integrating the whole variety of methods and means of teaching and monitoring the results of student educational activity.
The methodology for assessing the levels of the stochastic competence formedness in students of general upper secondary schools contains:
• a certain content area of the stochastic competence in high school students;
• diagnosable indicators;
• traditional and innovative methods of control and measuring instruments that are distinguished by accuracy and reliability;
• specified conditions that promote manifestation of competences as a result of teacher monitoring and measuring activities;
• designed monitoring test materials;
• mechanisms for evaluating the academic performance of a class through a cumulative performance recording system that allows for determination of the level of the stochastic competence formedness at the final stage of instruction.
Successful competence measurements are achieved through integrated application of both traditional control means and methods and the means of information and remote learning technologies. Some are used to assess the knowledge component (test papers, final tests, etc.), some other – for the motivational component (the use of interactive modules, computer models), and the others – for the activity component (real-world problems, non-standard tasks, competence-oriented tests, etc.).
With reference to the limited content of stochastic elements in the upper secondary school curriculum in mathematics at the level intended for STEM majors, to ensure extension, generalization and universality of high school students’ knowledge in the discipline under study, the authors have developed an academic course in stochastics. It acts as a means to deepen and extend knowledge on combinatorial analysis, the theory of probability and mathematical statistics; serves as an impetus for students to understand the presence of an activity that directly depends on the complex laws of probability theory in all the public life spheres; meets the requirements of educational standards (Kerimbayev et al., 2017: 59).
The implemented training course aims to correct, extend and deepen the basic stochastic knowledge of students received in the middle school.
Figure 2
The training course from the website Stochastic.ru
The training course was uploaded to the website Stochastic.ru (Figure 2). It acted as an educational environment conducive to autonomous universalization and deepening of knowledge on the theoretical and practical foundations of stochastics. The course allows for:
Thus, the performance evaluation has made it possible to successfully implement an integral system of both methodological and information means that facilitate elucidation of interrelated issues and selection of the best substantiated forms and methods for their implementation. Since distribution of stochastic methods is so universal for academic subject areas, it is advisable to take into account, deepen and synthesize the entire range of stochastic influence in order to increase the overall efficiency and effectiveness of the educational process.
Measurements of the level of the stochastic competence formedness in high school students over a certain observation period have shown the following results (Table 1):
Table 1
Measurements of the level of the stochastic
competence formedness in high school students

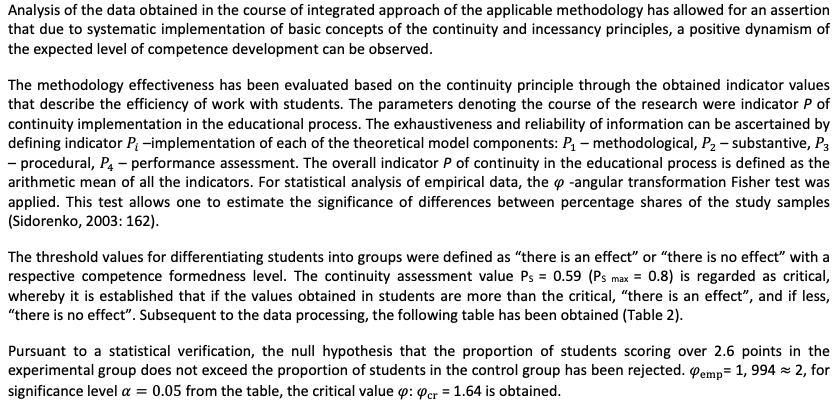
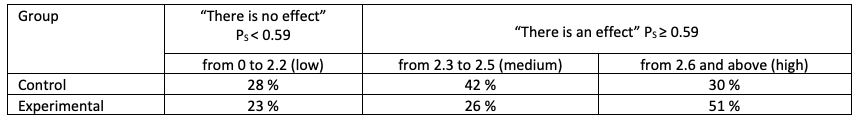
Table 2
Assessment of reliability of differences between sample percentages

Summarizing the results, one obtains that the principle of continuity, based whereon the method of measuring the levels of the stochastic competence formedness in students of 10th and 11th grades has been developed, has become the major success factor, including through a comprehensive approach to the implementable methodology. According to the authors, the essential feature of the methodology for improving the stochastic teaching process organization in general upper secondary school that has positively influenced the course of the entire educational process is as follows:
conformity with the basic framework of the continuity principle has led to a more accurate and profound acquisition of stochastic knowledge; to identification of underlying genetic affinity that makes it possible to apply the knowledge acquired by students in other subject areas; development of the real-world problem solving skill; gaining experience in developing stochastic models of the objects under study;
from the perspective of an integrated approach, the skill to expertly navigate within the information space has developed; the use of information technologies has helped to avoid verbatim learning of the conceptual material;
integration of stochastic methods and information technologies has led to an increase in cognitive interest and motivation of students to the subject learning, a broadened scientific horizon, an organized complex mental activity; it has served as an impetus for raising the overall level of math culture (hence, stochastic one too) and to unlocking the “inner” potential of a student’s personality.
Socio-political changes in society assign a special part to education raising the standards for future graduates. The knowledge, skills and expertise of students in schools should help them to rapidly adapt to demanding requirements imposed by the state and the social mandate. Education is not only considered as a result of acquiring knowledge, but also as a process of transformation and application of this knowledge, that is, systematic mastery of competences, including stochastic ones. The possibility to fulfill this condition is fully actualized in the implementation of continuity, which also contributes to the incessancy of instruction.
The current processes in Russian education prove that the continuity mechanisms are very poorly represented. Therefore, the lack of an integrated methodological system for continuous formation of basic stochastic concepts, skills and expertise is explicitly unconducive to the holistic perception presented in the school course in mathematics and stochastic material.
The didactic value of the proposed concept for successful implementation of continuity mechanisms in the system of school mathematical education is that high school students have shown a high performance in terms of possessing stochastic knowledge, expertise and skills of proper comprehension and analysis of information, solving real-world problems depending on their own experience, the ability to draw accurate conclusions and interferences.
In the process of determining the levels of formed stochastic competence, it was established that the students properly understood the content of probabilistic problems, were aware of the fact that a large number of various contingencies could be substantiated by knowledge of probabilistic regularities.
The presented concept ensures the continuity of school mathematical education, leads to the stochastic competence formation in future graduates of general education schools, will smooth the transition from general school education to higher or secondary vocational education with further study of mathematical disciplines. The prospects for further research development are extremely multifaceted, while the educational potential of the teaching and assessment methods and means applied on the basis of an integrated approach, whose major factor is continuity, is enormous and should be further improved.
The study was conducted with the financial support from the Russian Foundation for Basic Research (Department of Humanities and Social Sciences), Project 17-36-01004 “Theoretical and methodological foundations for continuity and incessancy implementation in furtherance of the stochastic line in the school course in mathematics within the framework of system activity approach”.
Arkhipova, S.V. (2009). Continuity in education: the sociological analysis. PhD Thesis in Sociology: 22.00.06, Ekaterinburg.
Asmolov A.G. (2016). Continuous Education: Risks and Values of Russia in Conditions of Uncertainty. The Federal Directory. Education in Russia, 11, 19- 21.
Bunck, M.J.A., Terlien, E., Van Groenestijn ,M., Toll, S.W.M., Van Luit, J.E.H. (2017). Observing and analyzing children’s mathematical development, based on action theory. Educational Studies in Mathematics, 96(3), 289-304. Doi: 10.1007/s10649-017-9763-6
Bunyakovsky, V.Ya. (1846). Foundations of the mathematical probability theory: Compositions of V.Ya. Bunyakovskiy. St.Petersburg: Printery of the Imperial Academy of Sciences.
Byvsheva M.V. (2012). Succession Problem in the Context of Continuity of Educational System. Educational Strategies, 3, 28-31.
Concept of the development of mathematical education in the Russian Federation. (2013) https://onedrive.live.com/?cid=A4174044B6A3047D&id=A4174044B6A3047D%211493&parId=A4174044B6A3047D%211483&o=OneUp
Godnik, M. (1990). Continuity of upbringing and educational activities in conditions of continuing education. In Gershunsky B. (Ed.) Prospects for the development of the continuing education system. Moscow: Pedagogika.
Gruzdev, P.N. (1940). Pedagogy: a textbook for teacher training higher education institutions. Moscow: Uchpedgiz.
Holmberg, J. (2017). Applying a conceptual design framework to study teachers’ use of educational technology. Education and Information Technologies, 22(5), 2333-2349. Doi: 10.1007/s10639-016-9536-3
Kerimbayev, N., Abdykarimova S., Akramova A., Kultan J. (2017). LMS moodle: distance international education in cooperation of higher education institutions of different countries. Education and Information Technologies, 22(5), 2125-2139. Doi: 10.1007/s10639-016-9534-5
Kharisova, L.A. (2017). Continuous general education: problems and factors of development. Bulletin of Adyghe State University. Series 3: Pedagogy and Psychology, 1(193), 57-61.
Khinchin, A.Ya. (1938). Two problems from the probability theory! Successes of Mathematical Sciences, 5, 231-232.
Kitaeva, I.V. (2017). The formation of the stochastic competence of students in the study of mathematics with the use of interactive methods and teaching aids: the author's abstract of PhD Thesis in Education: 13.00.02. Yelets.
Kolmogorov, A.N. (1938). On analytical methods in the probability theory. Successes of Mathematical Sciences, 5, 5-41.
Kyveryalg, A.A., and Mikhailov, Z.E. (1986). Continuity as a teaching principle in secondary vocational schools. In Kirsanov A. (Ed.) Principle of training in a secondary vocational school: Collected scientific works. Moscow: APN USSR, pp. 70-78.
Ogorodnikov, I.T., and Shimbirev, P.N. (1950). Pedagogy: Textbook for teacher training institutes. 2nd ed., Moscow: Uchpedgiz.
Oleinik, P.I. (1974). The problem of the continuity of the labor training of pupils of a school and a technical school: the author's abstract of PhD Thesis in Pedagogy. Moscow.
Oreshkina, A.K. (2003). Continuity of the educational process in the system of continuous professional education. Life-long Education: Continuing Education for Sustainable Development, 192-195. https://cyberleninka.ru/article/n/preemstvennost-obrazovatelnogo-protsessa-v-sisteme-nepreryvnogo-professionalnogo-obrazovaniya
Payson, T.P. (2007). On the principle of continuity. Proceedings of the tenth regional conference on mathematics MAK-2007, Barnaul: Altay State University Press, pp. 157-158.
Pistrak, M.M. (1934). Pedagogy: a textbook for Higher Teacher Training. Moscow: Uchpedgiz.
Prosvirkin, V.N. (2010). Technology of continuity of the educational institution. Modern Preschool Education. Theory and Practice, 4, 43-45. https://cyberleninka.ru/article/v/tehnologiya-preemstvennosti-obrazovatelnogo-uchrezhdeniya
Revtovich, V.N. (1987). Continuity in teaching students of the preparatory department and university students: PhD Thesis in Pedagogy: 13.00.01. Minsk.
Shor, E.V. (1977). In the world of incidents. Chisinau: Cartia Moldoveniasca,
Sidorenko, E.V. (2003). Methods of mathematical processing in psychology. St. Petersburg: Rech Ltd.
Sitdikova, D.Sh. (1985). Didactic conditions of continuity in the forms and methods of training in secondary and higher schools: PhD Thesis in Pedagogy: 13.00.01. Kazan.
Slutsky, E.E. (1960). Selected works. The Probability Theory. Mathematical Statistics. Moscow: Publishing House of the USSR Academy of Sciences.
Smantzer, A.P. (2013). Theory and Practice of Implementing Succession in Teaching Schoolchildren and Students. Minsk: Byelorussian State University Press.
Volk, M., Cotic, M., Starcic, A.I., Zajc, M. (2017). Tablet-based cross-curricular maths vs. traditional maths classroom practice for higher-order learning outcomes. Computers & Education, 114, 1-23. Doi: 10.1016/j.compedu.2017.06.004
Zainichev, R.M. (2015). Implementation of continuity in mathematical education: monograph. Naberezhnye Chelny: Naberezhnye Chelny State Pedagogical University Press.
1. Doctor of Sciences (Education); Bunin Yelets State University, Yelets, Russian Federation. Contact e-mail: Scherbatych2017@yandex.ru
2. Magistr; Bunin Yelets State University, Yelets, Russian Federation